Microscopes can be very simple or very complex devices – ranging from the classic magnifying glass to million-dollar scanning electron microscopes used in the most advanced labs on the Earth. Numerical aperture is an important concept in understanding how light microscopes resolve images and how you can avoid empty magnification.
In microscopy numerical aperture is the ability of an objective lens to collect and accept incoming light condensed into a cone of light from the condenser. The numerical aperture inscribed on the side of the objective represents the numerical aperture required for optimal resolution.
It is easy from a high-level point of view to know how microscopes work. But how do these individual components work? How are lenses designed to work with the incoming light? How does the eyepiece focus all the light correctly? Let’s find out!
Lenses
Lenses are.. everywhere! If you are wearing glasses to read this article, you are using lenses. Your car mirrors, your laptop screen, camera, etc.
They are pretty intuitive – glass (or some kind of see-through plastic) is bent and shaped such that it changes how light from the other side reaches the side your eye is! But they can be very VERY complex to actually design, analyze, and engineer.
It is definitely not as easy as bending glass a certain way. There is a lot of technical terminology, but we’ll focus on one key parameter of any lens: it’s Numerical Aperture or NA.
How Do Lenses Work?
First, we need to look at and think about what lenses do. A schematic always helps. So let’s take the classic convex (think: bump out) lens with an object and light source:
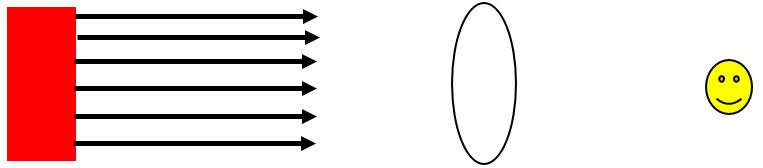
So we have these incident light rays (the arrows) coming towards the lens. The concave lens focuses things “inwards”:
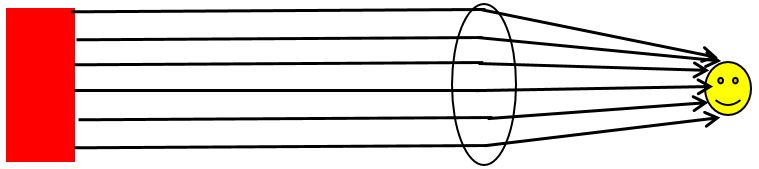
How about if the smiley is closer to the lens?

This all makes sense, right? If the object is closer, there is less path the light has to travel, and the light looks “fatter”. If it is further away, the light needs to travel more so it gets “narrower”.
We need to characterize this somehow, but the convention is that we can’t really use distance. We want to use angles. Let’s look at just the topmost and bottom most beams:
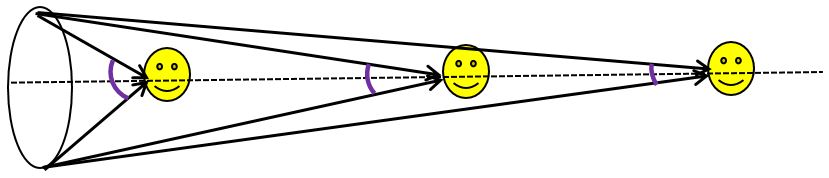
That purple line in-between the rays is the angle between the lanes. Notice how as the object gets closer to the lens, the purple line, hence angle, gets bigger. This is part of the definition of numerical aperture!
Every time you are viewing something through a microscope, light will converge on the object. This gathering of light to the object results in a “cone” shape with a defining angle for the cone. And notice something else about the first three images – particularly notice what the spacing is for the light at the object.
If the smiley is close to the lens, there is more spacing in between the light rays at the object. If it is further away, the light rays end up all on top of each other. This is a key fact! Remember light is what we are using to probe the object. The more “time” and “space” the light has to interact with the object – the more details the light “gathers” and hence our image is more clear!
To sum up real quick before the finale:
- Light needs room to “bounce around” the object in order to get information about it.
- The more “bouncing around” it does – the better our image.
- If the object is close to the lens, more light gets to bounce around the object.
- If the object is far from the lens, light ends up “on top of each other” and less of the object gets probed.
- We define the “closeness” of the object not by distance, but by the angle at which a cone of light is drawn from the lens to the object.
Smaller angle – worse quality. Larger angle – better quality. This is what we define as Numerical Aperture. It is a quick, single number that describes “hey, for a given lens, how well does it gather light from the object we want to see? The “how well” part is described by the angle. And we have a formula for this – numerical aperture is shorted to NA:
NA = n x sin(Ө)
Sin is the math function, that big letter in the parentheses is the angle, and… what is n?
n is the index of refraction. We focused on how light hits the object and comes back – but how it travels from the object to the lens is important.
Usually, microscopes are used in air – where n = 1. Does light really change its path when it goes through plain air? No! But what happens if this microscope was underwater?
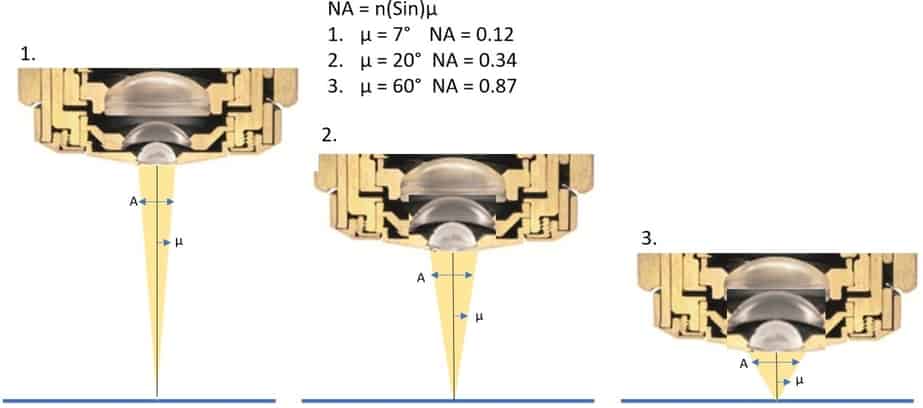
What if the air was really dirty air, where dust and dirt might affect the path of the light? Therefore, we need this factor of n. n describes the medium (think: environment) the light is travel to and from. NA is limited in between values of 0 and 1. There can never ideally be an NA of 1 with air as the medium, but values of around 0.95 are achievable.
Design of lenses and Numerical Aperture
We got the basics covered. So what does it matter? Well lenses (as described in the intro) are used for so many applications. Take the easiest one – if you are near or far sighted. You have a lens specifically made for you because your eye is unique. This lens will be constructed to correct your eyes, and this lens will have a designated NA. But can you just take that lens out of your glasses and put it in a microscope? Of course not! Lenses are tailored to their specific application.
So do all microscopes have the same numerical aperture? I mean, aren’t they all trying to magnify something to the best of its ability? Again – it’s a complicated answer. Say you are trying to study multiple kinds of materials. Each one of these materials can best be viewed using a certain kind of light. Maybe a blue laser or red laser.
There are also a lot of kinds of light with different shapes and propagation through space. Will one lens work for both of these? Most likely not! You need to tailor your lens to your specific application. If you have multiple lenses laying around, looking at it’s NA and other specifications help you – the scientist – decide if it is the correct one to use.
When to use High and low Numerical aperture
The numerical aperture of a lens also determines how bright you can observe the object you want to see. Perhaps the object is very sensitive to light. In this case, it might be beneficial to sacrifice some resolution to ensure you don’t damage the precious sample you wish to see.
Higher numerical apertures are used with higher power objectives so as a general rule the higher the magnification the higher the numerical aperture. The numerical aperture is controlled by using the lever on the iris diaphragm. Opening the iris diaphragm will raise the numerical aperture and closing the iris diaphragm lowers the numerical aperture. As stated above, for best results the numerical aperture of the objective currently set must match the numerical aperture setting on the iris diaphragm apparatus. Some condensers will have the numerical aperture marked and others will not. One without markings will require a bit of trial and error.
Improvements of the Numerical Aperture of lenses
Lens and optics have been around for centuries, so the general construction of how lenses are built to focus onto an object isn’t going to change so much. It is a well established engineering solution. But that doesn’t mean engineers and scientists aren’t looking for ways to improve numerical apertures of lens systems. As we talked about earlier – there is this factor of “n” in the formula for numerical aperture:
NA = n x sin(Ө)
If we increase n… numerical aperture should go up! This is what we want. The typical environment we work in is just plain air (n~1). But what if we change the environment to a liquid or oil (n=1.33)? Numerical aperture will go up and we can improve the image we see!
The use of immersion oils is something scientists are actively using today achieve numerical apertures greater than 1 with high powered objectives. These objectives slowly drip water onto the sample and lens so that light is confined in it. This increases “n” and increases the resolution.
These types of lenses are expensive (sometimes 10x the normal price of lenses, made by companies like Zeiss, LOMO, Nikon) but can very much improve the quality of a microscope.
Takeaways
In summary the numerical aperture of a lens is the ability of a lens to collect and accept incoming light. It is dependent on two values: the angle of where the objective is and the edges of the incoming light, and the medium the light is traveling through.
Numerical aperture is one of the most important specification in any microscope system as it really quantifies the “quality” of how well the image can be captured. I hope this article has helped you to understand a complex topic in optics and microscopy.
